When I studied Euclidean Geometry, I was unaware and often confused by all of the different centers of a triangle. So first, I am going to explain how to find each of these centers, and then explain Euler's line that he discovered.
First, we will look at the orthocenter. The orthocenter, point D, is where the three altitudes of the triangle intersect as shown below.
Next, we have the triangle centroid, point G below. This center is where the three medians intersect. The median is found by finding the midpoint of each side of the triangle and connecting the midpoint to the opposite vertex.
The circumcenter, point H below, is the point where the perpendicular bisectors of each side intersect.
Another center of circle, de Longchamps point, point K below, can be found. De Longchamps point is found by reflecting the orthocenter over the circumcenter as shown below.
Finally, we will look at the center of Euler's circle which is also known as the nine-point circle. This circle is found by connecting nine points together. You connect: the midpoint of each side of the triangle, the foot of each altitude, and the midpoint of the three segments that connect the orthocenter to the vertices. The Euler circle is shown below with center at point S.
The pink line above is the Euler line passing through the orthocenter(point D), the triangle centroid (point G), the circumcenter (point H), the de Longchamps point (point K), and the center of Euler's circle (point S). Since this image is quite complex with all the lines you need to find these centers, I simplified it below.
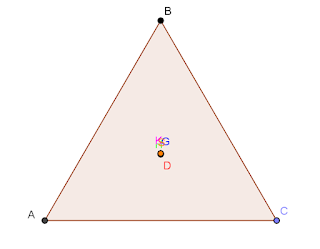
The only triangle that does not contain Euler's line is an equilateral triangle. For equilateral triangles, all the centers of the triangle fall on exactly the same point. Thus, you cannot create a line connecting all these points when there is only one point.
I think that realizing that Euler's line holds true in all triangles except equilateral triangles is quite incredible. When I first learned about it I was amazed. It made me wonder how many of these types of discoveries in geometry I don't know about yet or have not yet been discovered.
Also, if I ever become a geometry teacher, I would definitely introduce my class to Euler and his line and circle. I think these concepts would grasp students interest and make them more excited to learn about geometry.







After reading this post I believe that I have a better understanding of Euler's line and Euler's circle. One is able to follow this post since there is a step by step process in which you take with explaining Euler's process with his discovery. From my experience, Geometry within high school wasn't as informative as it could have been and implementing concepts such as Euler's line or Euler's circle is one of the ways to make Geometry more interesting to students. It seems as if Mathematics will always be a subject in which we can grow and find new connections. I believe that as we progress through time we will make more and more discoveries connecting to Euler. One suggestion that I would have would be a more in-depth explanation on how it would be good to implement at a high school level or even younger. Overall though a really good post!
ReplyDeleteAbby, I love this blogpost! I too was a little confused when this was discussed in Euclidean. I have a much better understanding now. I like the way you illustrated your point and used geogebra. I like how you tie education into this post. Do you think all students should be familiar with geogebra and how do you tie in Euler if it is not apart of the standards? I'm wondering that in my own teaching. Overall, this is a really thorough post and I enjoyed reading.
ReplyDeleteStrong post. Clear exposition of the ideas (content and complete), nice consolidation. The only thing I might add is a sentence for each center about how it is the center. You say how to find it, but why should we think of the de Longchamps center as the middle of a triangle?
ReplyDelete5Cs+
ps. if that's your GeoGebra, you could put it on the tube and link it.